



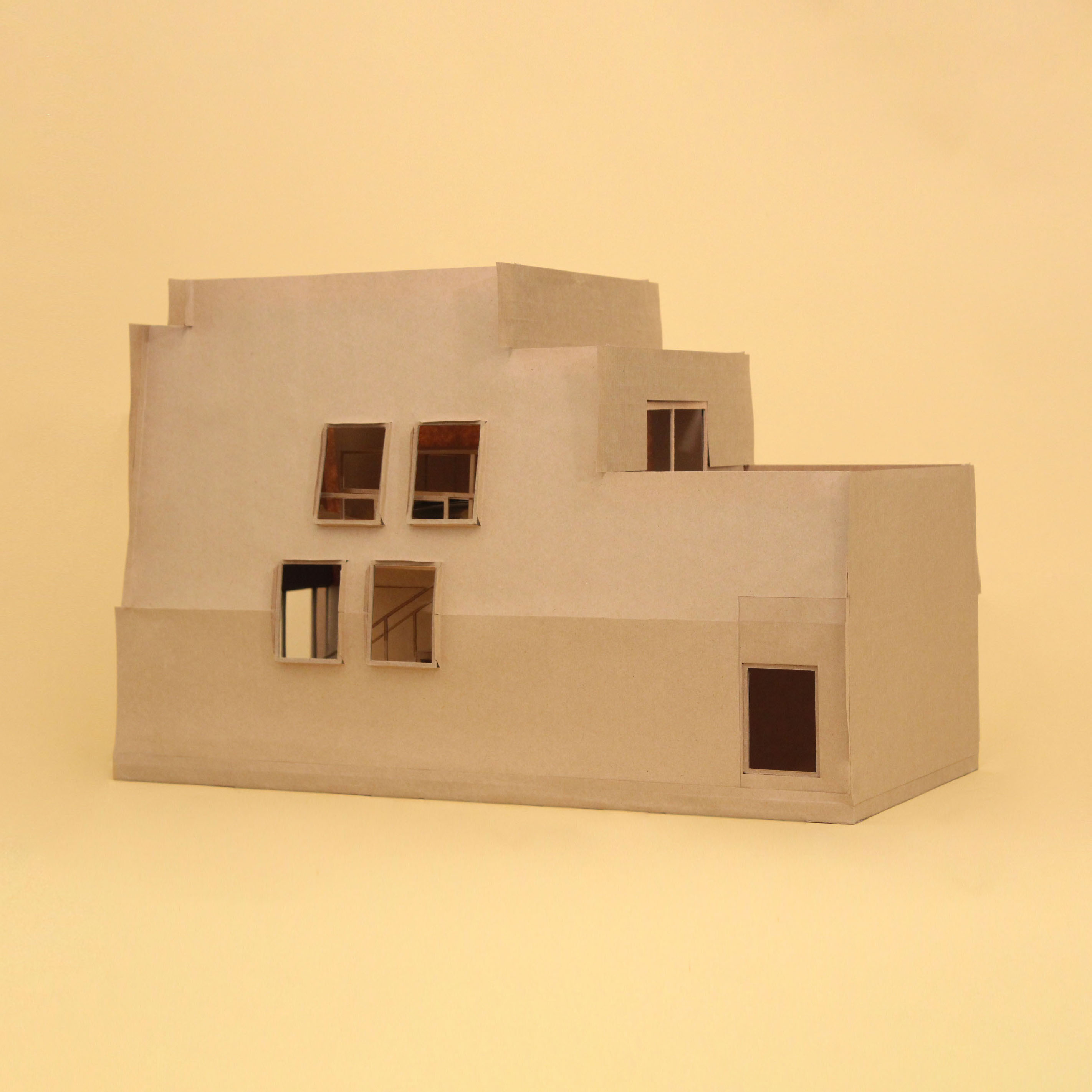
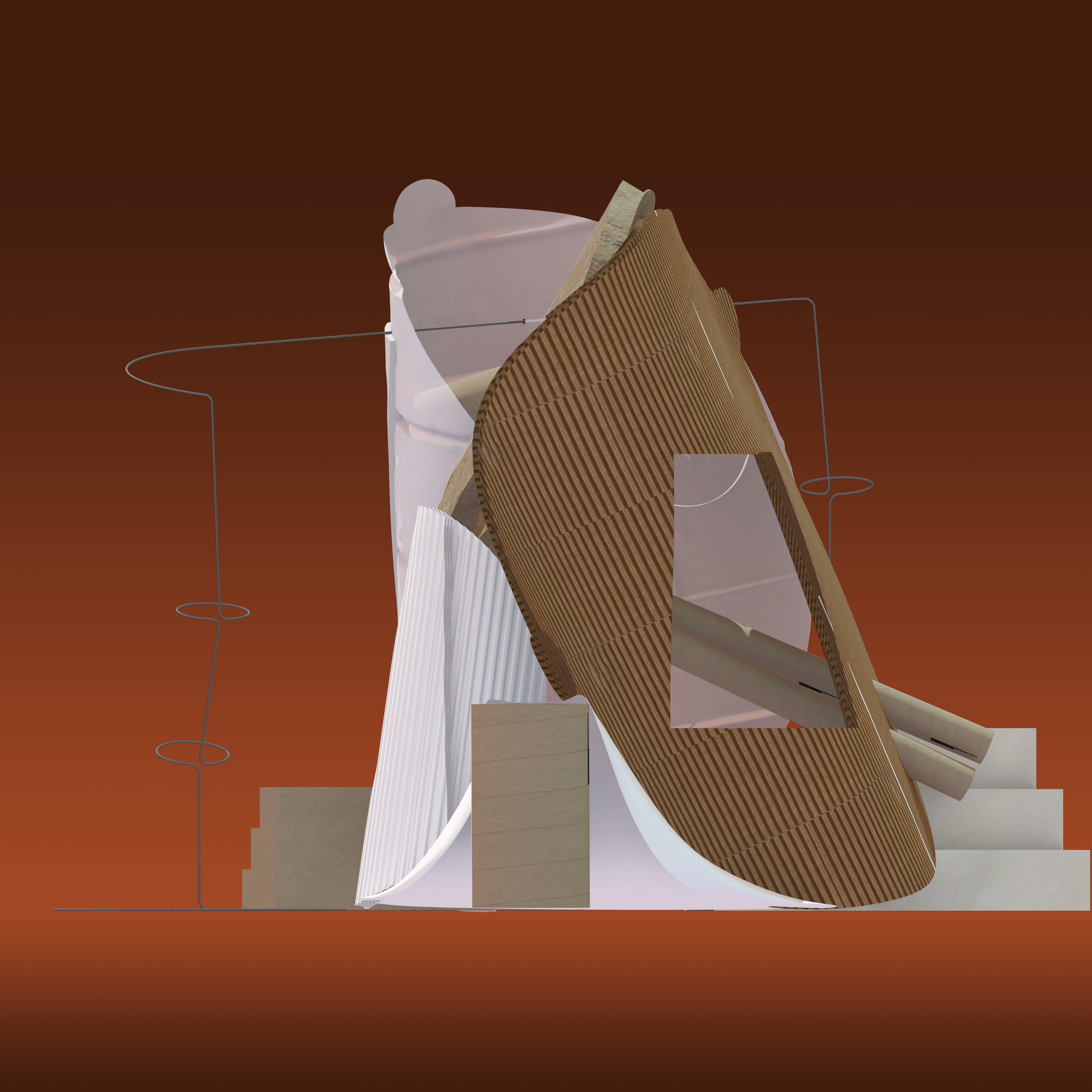

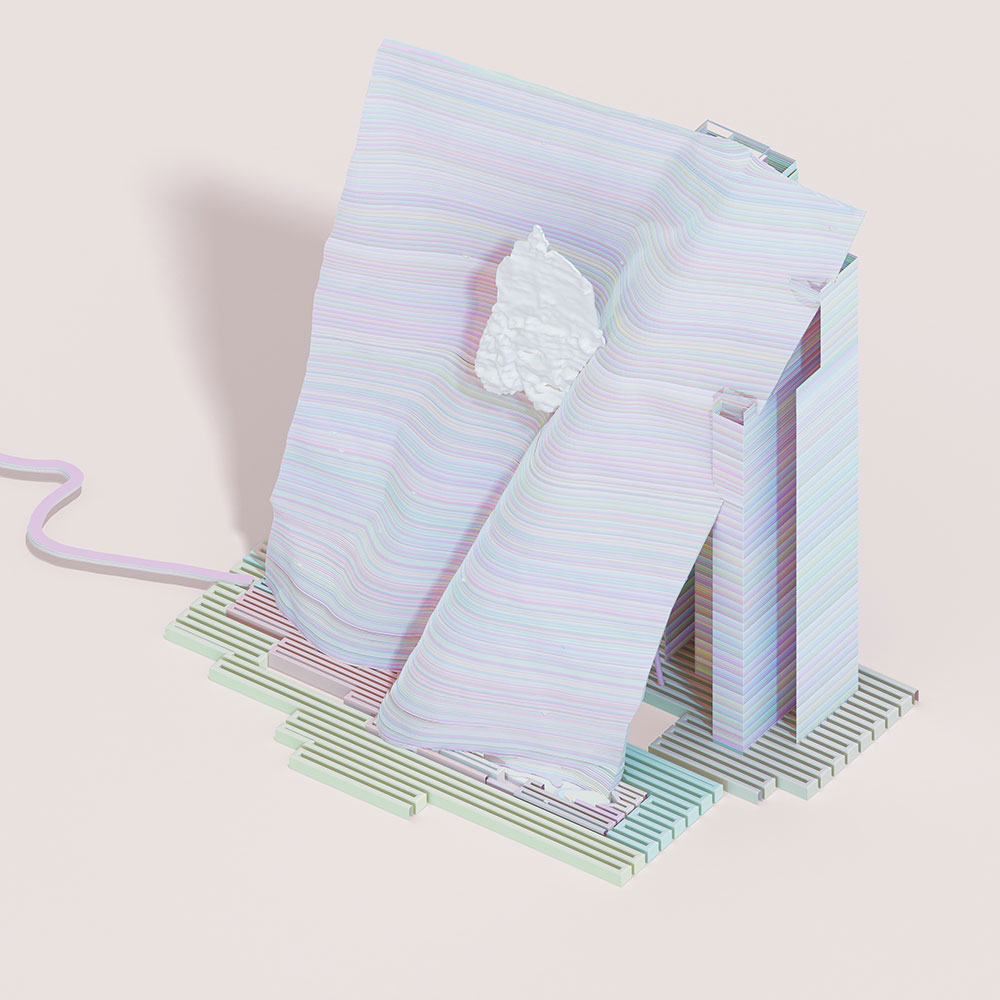
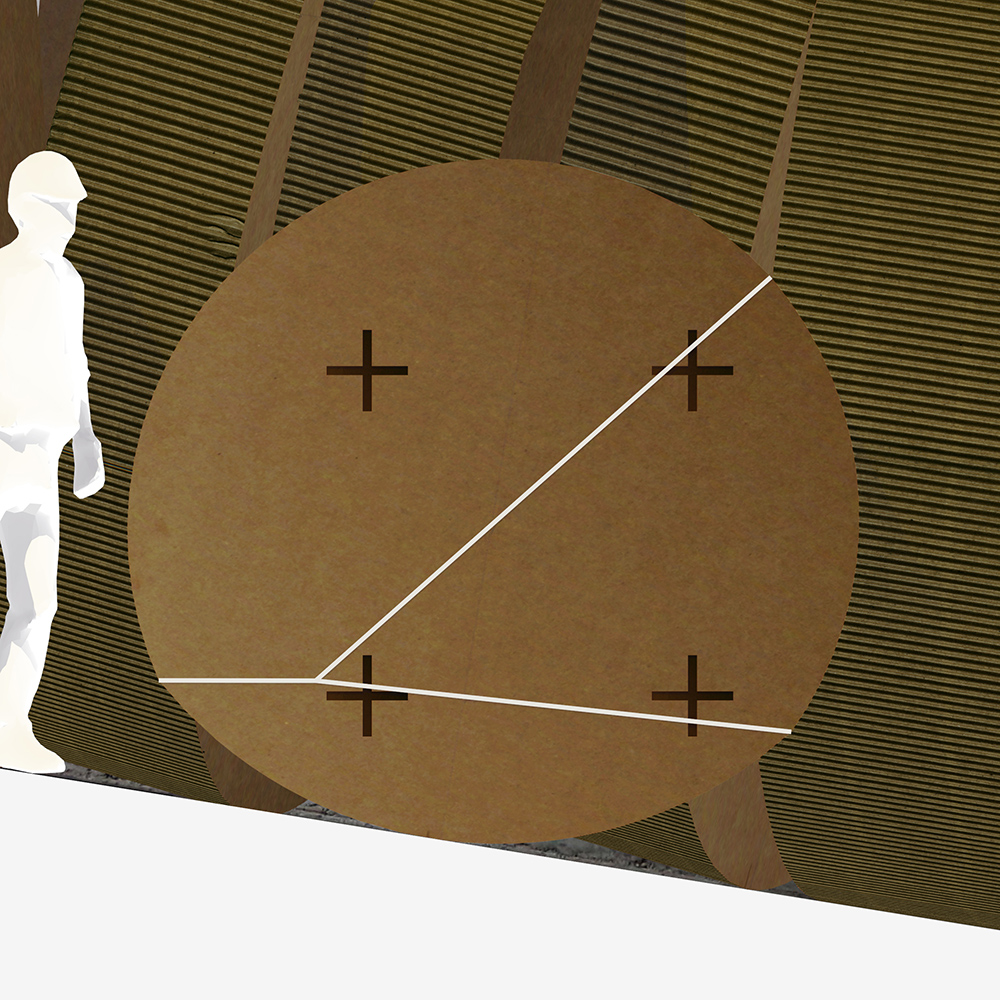











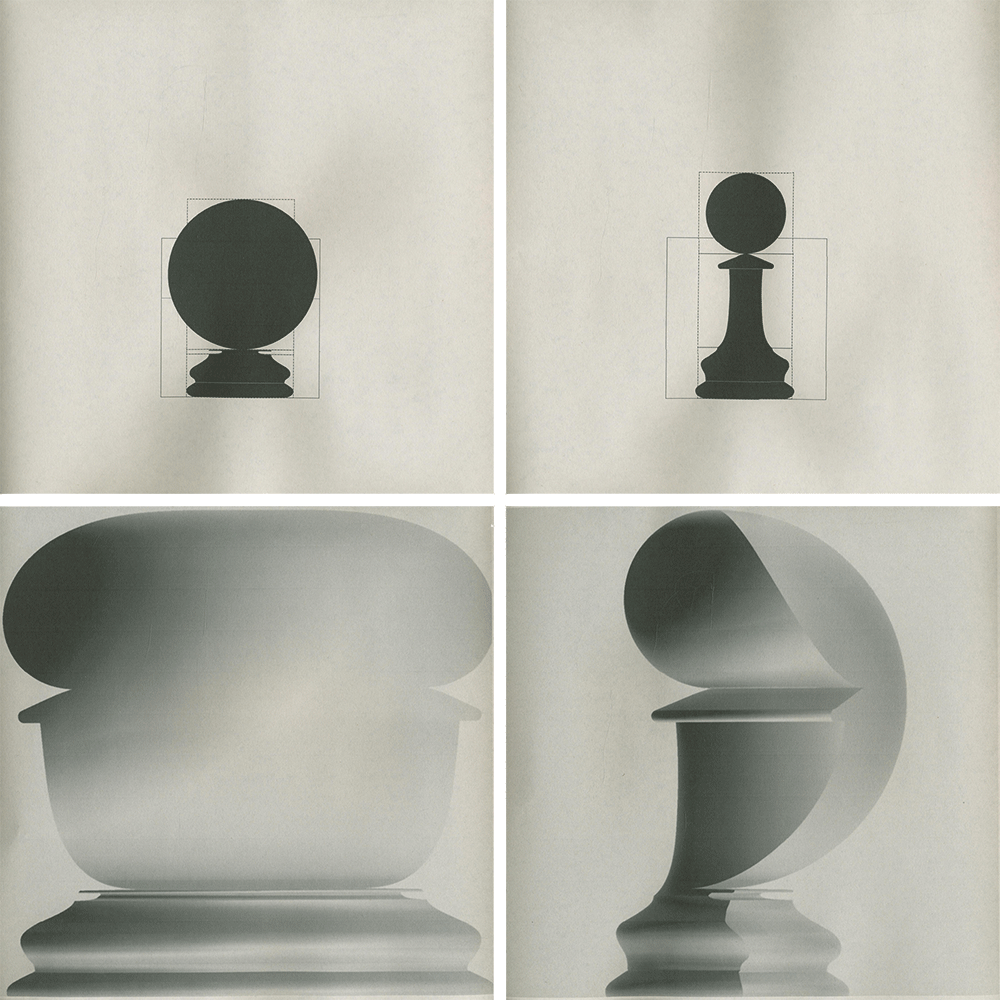

d.esk is a practice that makes and learns and experiments and fails, over and over and over again. It uses paper, compares things, and designs buildings through open-ended inquiry and client-based projects. Our work begins with long standing conundrums or assumptions about how architectural form is conceived, and we then tease out techniques for alternatives. We choose tape over glue, tone over hue, coherence over style, abrupt over transitional, creativity through convention, soft and hard, things provisional over things fixed, and over or, strange over weird, subtle over obvious, considered over neglected, now over new, cheap as luxury, and alliances over commitments. We value good taste, deep thought, and ethical imagination.
The practice is led by David Eskenazi (he/him) in Los Angeles, with current projects in California and Mexico. Alongside the practice, David writes and teaches, with publications in journals including Log, Project, Offramp, and Pidgin. He’s been awarded the League Prize from the Architectural League of New York, the Oberdick Fellowship at the University of Michigan, the LeFevre Fellowship at the Ohio State University, and was a MacDowell Fellow. Currently, David is a design studio and visual studies faculty at SCI-Arc.
The practice is led by David Eskenazi (he/him) in Los Angeles, with current projects in California and Mexico. Alongside the practice, David writes and teaches, with publications in journals including Log, Project, Offramp, and Pidgin. He’s been awarded the League Prize from the Architectural League of New York, the Oberdick Fellowship at the University of Michigan, the LeFevre Fellowship at the Ohio State University, and was a MacDowell Fellow. Currently, David is a design studio and visual studies faculty at SCI-Arc.
Paperweight
![]()
Afterscale Devices




